These features can be accessed through Cross-Sectional Analysis from Data Analysis on the Main Menu of IFs.

Extended Option. When you use the input line for independent or dependent variable selection, it is also possible to compute a variable as a simple function of two or more other variables (e.g. as the sum, difference, quotient, or product of two other variables).

Now touch the Plot button to create a scatter plot showing the relationship between the two selected variables for as many countries as exist in the data set. You will see that life expectancy increases rapidly as GDP per capita rises. The scatter plot may be sufficient for many users of IFs. You can print the scatter plot or save it for other analysis or for use in other applications.

Some users, however, will want to proceed further and to describe the relationship between the two variables with an equation. If you have Microsoft Excel available on your computer, you can do this by touching the Excel button from the Scatter plot. That button actually creates a link with Excel and carries the scatter plot (with supporting data) to Excel for further analysis.

Excel opens up many additional features for your use that can be described in more detail using Excel’s Help menu. But let’s walk through the process of fitting a line and associated equation to the data (important for the next Lesson). First, maximize the Excel screen by using the maximize button in the upper right hand corner of the window (the icon of a single large window).

Then click anywhere on the graph to activate it. That allows you then to right-click on various components of the graph in order to edit those components. For example, right-click on the straight line that Excel has fit to the data. You will see a small pop-up menu with a couple of options. Select the Format Trendline option and you will see the following window.

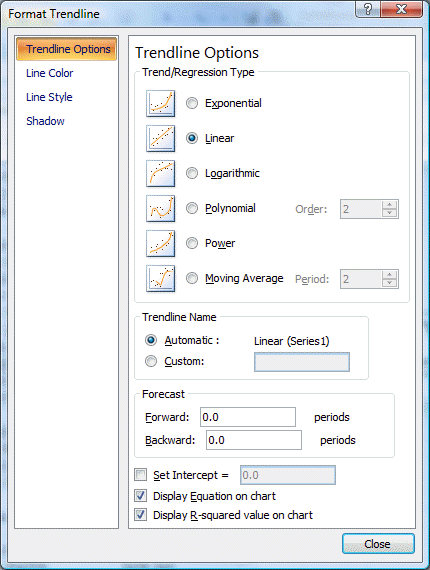
Choose the Type tab and select the logarithmic trend/regression type for a better fit.

Then choose the Options tab for additional choices including checking boxes for "Display Equation on Chart" and "Display R-Squared Value on Chart." Although both options are checked, the equation and R-Squared are sometimes not initially visible on the chart because they are not placed properly when you open Excel (it depends on the variables you plot). If they are not visible, turn the options both off, close the Format Trendline window, right-click on the line, select again the Format Trendline option, and again select the Options folder. Turn the Display Equation and Display R-Squared options back on and exit from the Format Trendline window again. You should now see the equation and r-squared someplace on the graph and can drag them to a better location, nearer the intersection of the two axis.

If you now right-click on the dependent or y-axis, you will see a small pop-up menu with several options. Choose the format axis option and the following window will appear:

Under the Scale tab you will find maximum value, minimum value, and other settings. Often you will find it very useful to reset maximum or minimum values. Try, for example, setting the minimum on the y-axis to 0 and the maximum to 100.

There is much more that you can do with Excel, including copying the figure you have just created and incorporating it into a word processing file. Experiment.

Exit from Excel (no need to save the file unless you want to), and click on Continue to move from the Scatterplot back to the Select Variables to Analyze Across Countries window (reproduced near the top of this lesson).

Although the introduction of a control variable works helps in looking at relationships involving up to three variables (dependent, independent, and control), it is possible to examine relationships involving as many as five independent variables (see again the IFs window shown earlier on analyzing variables across countries). The key to this is selection of the Statistics option that you can see at the bottom of that window (next to the Plot button). After selecting a dependent variable and as many as 5 independent variables, touch the Statistics button. That will display the set of transformation options.

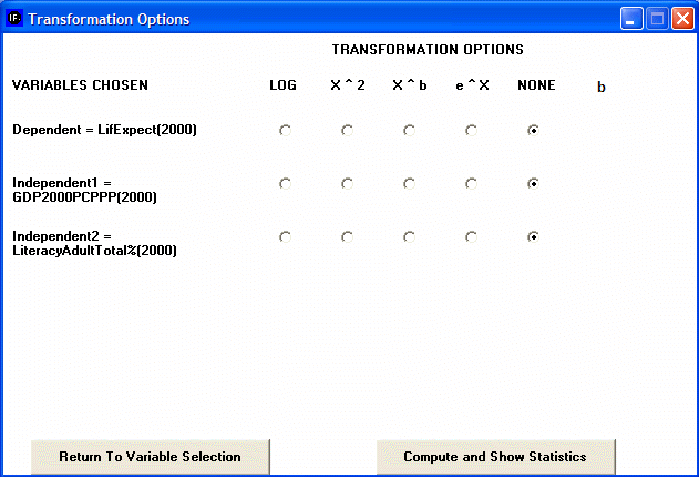
The transformation options allow you to apply transformations to your dependent and/or independent variables, just as you saw earlier in Excel that a logarithmic transformation of GDP per capita helped explain life expectancy better. Choose here again the logarithmic transformation of GDP per capita and then select the button Compute and Show Statistics. That will produce the following window showing statistics about the relationships among these three variables. These statistics will not be explained here – please see a standard statistics textbook.

Computed Functions. Click on the sub-option for computed functions to obtain the following window.

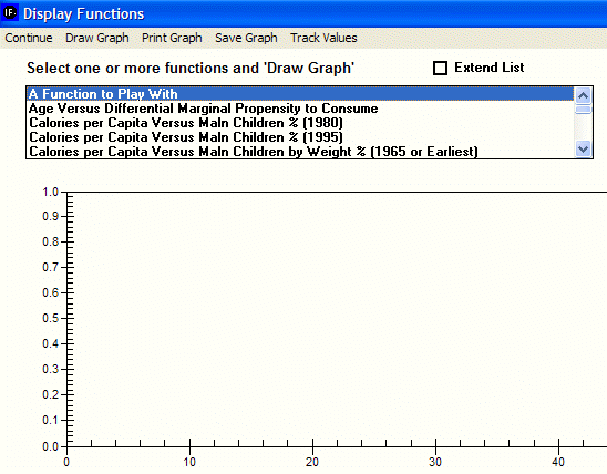
Many two-variable relationships have been computed within IFs using the cross-sectional analysis capabilities described earlier. For instance, relationships have often been computed for the same two variables at different points in time to gain insight about how the cross-sectional relationships have changed over time.

Click the Extend List box in the window above to see the list of computed functions more clearly. Then scroll down until you see GDP/Capita (PPP) Versus Life Expectancy (1962). Because of data availability this was the earliest relationship computed between the two variables. Notice that other relationships have been computed at intervals. Holding the control (Ctrl) button down click on the relationships between those two variables for 1962, 1980, and 1995. All three should be high-lighted and therefore selected. Now click on the Draw Graph option to create a graph that contains all three.

Note that at low levels of GDP per capita at PPP (in constant dollars over time) the life expectancy has generally gone up. In other words, countries have succeeded in raising life expectancy even at low levels of GDP per capita. Why? It could be some combination of improved medical technology and of improved health policy. In any case, the shift in the relationship over time suggests the value of multi-variate analysis of the relationship, not just bi-variate.

Another feature of this section of IFs is the Computations button.