The income share of the poorest 20 percent of the population (INCSHR) depends on the GDP per capita at PPP (GDPPCP) and on an exogenous income share multiplier (incshrm).

The introduction of different household types into the social accounting matrix structure of IFs made possible the computation of a more sophisticated measure of income distribution tied directly to the model’s computation of household income (HHINC) and household size (HHPOP) by type. A domestic Gini value (GINIDOM) is calculated from a function that uses the normal Lorenz curve foundation for Gini indices. Because that function can calculate values that are quite different from the empirical initial values, a ratio of the empirical value to the initial computed value (GINIDOMRI) is used for scaling purposes. The model’s formulation of the relative household income levels of different household types, and therefore the calculation of a domestic GINI based on those income levels, are in early versions and are still rather crude.

One value of a domestic Gini calculation is that it, in turn, makes possible the calculation of the percentage of population living on less than one dollar per day (INCOMELT1) or two dollars per day (INCOMELT2). Functions were estimated linking GDP per capita at purchasing power (GDPPCP) and the Gini index to those percentages. Again, IFs uses initial conditions for scaling purposes.

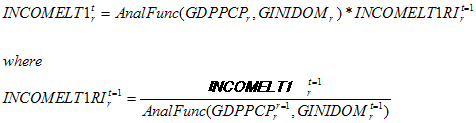
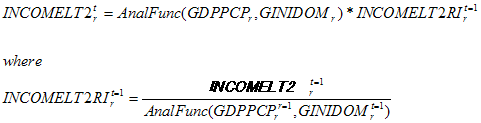
IFs also calculates a global Gini index across all countries/regions in the model, again using the standard Lorenz curve approach to areas of inequality and equality. It does not yet take into account intra-regional income differentials, but the foundation is now in place to do so.

The user interface of IFs now uses the same Lorenz-curve approach to allow the user to calculate a specialized-display GINI for any variable that can be represented across all countries/regions of the model.